بخشی از پاورپوینت
--- پاورپوینت شامل تصاویر میباشد ----
اسلاید 1 :
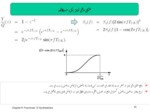
مثال: طیف مولفه های ناخواسته کسری
Let us first find the Fourier transform of one period of the waveform (from t1 to t2). This waveform consists of nine 990-ns cycles. If we had an infinite number of such cycles, the Fourier transform would contain only harmonics of 1.01 MHz. With nine cycles, the energy is spread out of the impulses. If this waveform is repeated every 10 μs, its Fourier transform is multiplied by a train of impulses located at integer multiples of 0.1 MHz. The spectrum thus appears as shown in figure below.
اسلاید 2 :
تصادفی سازی و شکل دهی نویز: تصادفی سازی ضریب
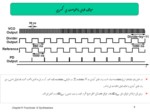
xFB(t) یک دنباله تصادفی از تناوب های990-ns و 1090-ns را نشان می دهد.
xFB(t) اکنون دارای مدولاسیون فاز تصادفی است:
تصادفی سازی ضریب، تناوب در رفتار حلقه را خواهند شکست و باندهای کناری قطعی را به نویز تبدیل می کنند.
فرکانس لحظه ای سیگنال پسخور به صورت زیر نشان داده می شود:
که در آن b(t) به صورت تصادفی مقدار صفر و یک را می گیرد و دارای مقدار میانگین α است.
می توانیم آن را برحسب میانگین و یک متغیر تصادفی دیگر با میانگین صفر بنویسیم:
اسلاید 3 :
مثال: تصادفی سازی
Plot b(t) and q(t) as a function of time.
The sequence b(t) contains an occasional square pulse so that the average is α. Subtracting α from b(t) yields the noise waveform, q(t).
If q(t) << N + α, we have
The feedback waveform arriving at the PFD
Phase noise given by:
اسلاید 4 :
مثالی از نویز فاز
With the aid of the wave form obtained last Example for q(t), we arrive at the random triangular waveform shown below:
The time integral of a function leads to a factor of 1/s in the frequency domain. Thus, the power spectral density of q(t) must be multiplied by [2πfout/(N + α)2 /ω] 2,
where Sq(f) is the spectrum of the quantization noise, q(t). Note that this noise can be “referred” to the other PFD input—as if it existed in the reference waveform rather than the divider output.
اسلاید 5 :
نویز فاز خروجی مولد فرکانسی در پهنای باند حلقه
Compute Sq(f) if b(t) consists of square pulses of width Tb that randomly repeat at a rate of 1/Tb
We first determine the spectrum of b(t), Sb(f). As shown in Appendix I, Sb(f) is given by:
where the second term signifies the dc content. Thus,
revealing a main “lobe” between f = 0 and f = 1/Tb
اسلاید 6 :
شکل دهی پایه ای نویز: تصادفی سازی باعث افزایش نویز فاز می گردد.
هدف تولید یک دنباله ی دودویی تصادفی , b(t), به گونه ای است که ضریب تقسیم کننده بین N و N+1 به نحوی تغییر کند که(1) مقدار میانگین این دنبال برابر α باشد, و (2) نویز این دنباله طیفی بالا گذر داشته باشد.
اسلاید 7 :
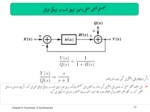
سیستم پسخور منفی با نویز تزریق شده در نزدیکی خروجی
اگر H(s) یک انتگرال گیر ایده آۀ باشد:
Øیک حلقه پسخور منفی که شامل یک انتگرال گیر باشد، به عنوان یک سیستم بالاگذر برای نویز تزریق شده در نزدیکی خروجی عمل می کند. اگر Q با زمان به آرامی تغییر کند، در نتیجه بهره حلقه بزرگ خواهد بود و W بسیار شبیه به Q میشود و بنابراین Y کوچک می گردد.
اسلاید 8 :
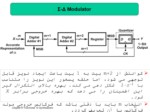
مثالی از نسخه گسسته-زمان سیستم قبلی(Ⅰ)
Discrete-time integration can be realized by delaying the signal and adding the result to itself. We observe that if, for example, A = 1, then the output continues to rise in unity increments in each clock cycle. Since the z-transform of a single-clock delay is equal to z-1, we draw the integrator as shown below and express the integrator transfer function as
اسلاید 9 :
مثالی از نسخه گسسته-زمان سیستم قبلی(Ⅱ)
Construct a discrete-time version of the system shown in the previous slide if H must operate as an integrator
Thus, the discrete-time version of the system appears as shown above. Here, if Q = 0, then
i.e., the output simply tracks the input with a delay. Also, if X = 0, then
This is a high-pass response (that of a differentiator) because subtracting the delayed version of a signal from the signal yields a small output if the signal does not change significantly during the delay.
اسلاید 10 :
افزودن سیگنال و نسخه تاخیر یافته آن برای فرکانس های بالا وپایین ساعت
اگر فرکانس ساعت افزایش یابد a(t) زمان کمتری برای تغییر دارد و a1 و a2 تفاوت کمی را نشان می دهند.